OMG, this is so fucking cool: Ancient engineering and Mayan counting

This week, I want to let the hyper-focus part of my ADHD run wild and each day write about something that is interesting to me. I don't know at the moment how this will turn out. I don't know if I'll write about something different every day, or write about the same topic each day this week, or even a combination of the two. It's just fells like it's been a few years since I've let this hyper-focus part of me go wild, and the blog posts I loved writing are the ones I could get super excited for.

I mentioned yesterday that I was made aware of a Mayan Culture lecture coming up near me in Moscow, and now when I start to think about it I want to talk about Mayans to anyone who will listen. Then I realized, I can just write it here! I get the possibility of an audience that might listen to me, and the sweet release of talking about something instead of leaving it bottled up inside.

Why do I find Mayan culture interesting? The very simple answer is that in the 6th grade, my teacher spent a month or so teaching us about the Mayans. Well, it wasn't as much as "teaching" as much as it was "having us watch 'The Second voyage of the Mimi' on VHS". Fun fact: that show stared a 14 or 15 year old Ben Affleck. And because I love you so much, here is an embed of the first episode on Youtube:

I currently have it on in the background while I work on this. Oh, pure old school PBS TV. I just don't even know how to describe it to people who never watched 80's PBS in person or reruns. It's too easy to pick on old shows though, and it did introduce me to a lot of interesting things. Like their engineering skills. They were able to build these super flat roads through the mountains. And they had  pyramids. These engineering feats are something that become interesting me now after spending sometime learning about Egyptian and Sumerian engineering.  Let me elaborate:

Egyptian architecture is the stuff of legends and is the topic of much discussion and conspiracy theories. Who built these ancient monoliths? A race of creatures from beyond the stars? It couldn't be that these structures stand as a monument to people wanting to push the limits of the world around them. Or it could, I guess. All those boring nerds called "archaeologists" have been taking the fun out of these things for years!

There is evidence of trial and error of pyramid building throughout the ages. The ability to build a pyramid didn't pop up over night, even if that idea sells books and TV shows to a mass audience. The construction of the pyramid of Giza is a wonder though, since they seem to display some understanding of math that was lost until the renaissance. Mainly the sides are slighty concave, said by a couple of people that they follow the curvature of the earth. Citation needed, since I'm finding some rumors of this this, but no actual good articles on it. This probably because of the knowledge we have of their math. . .

We do have some examples of Egyptian mathematics today! Sadly, they seem to be more basic problem sets aimed towards students. It does strike me as funny that homework has been around since the dawn of human history though. Thinking about Egyptian and Sumerian teenagers complain about homework brings a smile to my face. As much as I would love to talk about mathematics in these cultures, this is supposed to be about Mayans. I'll wrap this up by saying that while we have some mathematical texts from these cultures and, in the case of Mesopotamia, we have calculations, receipts, and such. But a study of the math of these cultures comes down to a study of the art and architecture.

Bringing it back to the Mayans, we have the structures, art and architecture to see examples of their knowledge of math. That famous calendar is something fascinating to look at. There is the geometry involved, the number system, and the understanding they had of astronomy. Astronomy and finance seem to be the breeding ground for mathematics. Probably because knowing when to plant crops and how to trade capital is two keys towards having a great civilization.

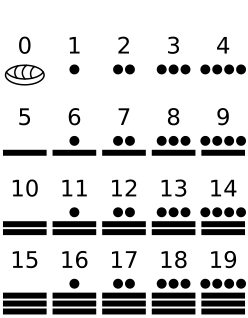The first part to understanding the architecture an the calendar is the counting system. The Mayan counting system has a special place in my heart because it was the system that helped me understand how counting systems work. All of us know how to count in base 10. Start at 0, count until 9, then at ten we add a new place. 1st grade math gives us the places - 10, 100, 1000, 100,000, 1,000,000 and so on. Add a new zero after 1 to get a new place. Some of us can count in binary, where 1 = 1, 10 =2, 100 = 4, 1000 = 8, 10000 = 16, and 100000 = 32. And some of us that can count in binary, can also understand hexadecimal. How do any of these work, and what do they have to do with the Mayan counting system? Well, in binary, or base 2, each new place has a value of 2^x, starting at the first place of 2^0. The first slot is the 1's place, and any number placed there can be multiplied by 1. Since we start counting at 0, and in base 2 we have only 2 numbers, we only have 2 numbers to put in the one's place - 0 or 1. 000001 = 1 * 1. The next number after 1 overflows into the next place, becoming 10. This next place is 2^1, or 2, and a number in that place can be multiplied  by 2^1 to find it's value. 000010 is 1 * 2^1, or 2 and for 000011 we get (1 * 2^1) + (1 * 2^0) to get three. I'll skip over hexadecimal for the moment, and talk about the Mayans. They have a base 20 system, which is easier to grasp than a base 16 system. In the one's place we have 20^0, then 20^1, 20^2, 20^3, and so on. To those of us not interested in figuring this out ourselves, that's 1, 400, 8000, then 160000 and up and up and up. Of course, they used their own number system as well, since the Arabic numbers never made it there. They had a place holder for the number zero, which is very impressive given the time period, and it's what allowed them to do place notation for their numbers. That's really impressive, but might be something to talk about later because talking about how hard it is to try and do calculations with roman numerals is whole discussion in itself.  As a take away from this: in 900 AD, the Mayans were using a number notation that wouldn't be used in Europe for another 600 years. With this system, they had ways of doing calculations beyond the algorithms of the Ancient Greeks. By which I mean that European mathematical texts were descriptions on how to use geometry in order to calculate things.

I'm stopping here, mainly because there's so much more to talk about and get excited about, but it's already long and dense. I want to get excited about the art and architecture of the civilization, and I want to spend a ton of time talking about the math of these people. If I don't stop myself now, then it will become a long rambling unfocused text. That's never good. So I'll put it out to anyone there to leave a comment about their thoughts, because there are a few things I didn't elaborate on, or didn't talk about, or maybe their's something you know that I don't. I would love to here your feed back.